Real-time QFT control for temperature in greenhouses
Main Article Content
Abstract
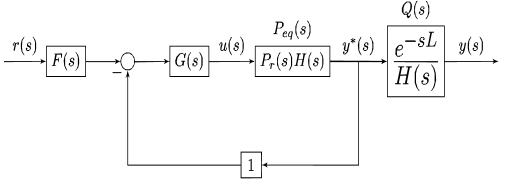
Sudden changes in a greenhouse environment negatively impact the development and production of crops, especially in greenhouses with natural ventilation when temperatures are low at night and change rapidly due to wet winds. A robust controller based on Quantitative Feedback Theory (QFT) from a Smith predictor structure for the dead-time system is proposed to mitigate these variations. This structure offers high stability based on the gain margin, the phase margin, and the rejection of disturbances in the system output. This design was contrasted with a PID controller based on performance indices according to the transient response and error in the presence of changes in the point of operation and charge disturbances. The final results showed that the dynamic response of the QFT controller improved compared to the PID controller results.
Downloads
Article Details

This work is licensed under a Creative Commons Attribution 4.0 International License.
Authors who publish in this journal agree to the following terms: Authors retain the copyright and guarantee the journal the right to be the first publication of the work, as well as, licensed under a Creative Commons Attribution License that allows others share the work with an acknowledgment of the authorship of the work and the initial publication in this journal. Authors may separately establish additional agreements for the non-exclusive distribution of the version of the work published in the journal (for example, placing it in an institutional repository or publishing it in a book), with acknowledgment of its initial publication in this journal. Authors are allowed and encouraged to disseminate their work electronically (for example, in institutional repositories or on their own website) before and during the submission process, as it may lead to productive exchanges as well as further citation earliest and oldest of published works.
How to Cite
References
[2] A. Visioli and Q. Zhong, Control of Integral Processes with Dead Time. London: Springer-Verlag, 2011.
[3] Z. D. Tian, “Algorithm and Implementation of Smith Predictive Control,” Appl. Mech. Mater., vol. 687–691, pp. 60–63, Nov. 2014.
[4] E. H. Gurban and G.-D. Andreescu, “Comparison of modified Smith predictor and PID controller tuned by genetic algorithms for greenhouse climate control,” 2014, pp. 79–83.
[5] S. A. C. Giraldo, R. C. C. Flesch, and J. E. Normey-Rico, “Multivariable Greenhouse Control Using the Filtered Smith Predictor,” J. Control Autom. Electr. Syst., vol. 27, no. 4, pp. 349–358, Aug. 2016.
[6] C. Esparza, R. Núñez, and F. González, “Model Reference Adaptive Position Controller with Smith Predictor for a Shaking-Table in Two Axes,” in Advances in Computational Intelligence, 2012, pp. 271–282.
[7] J. M. B. García, A. A. García, and E. F. Amorós, Electrónica de potencia: teoría y aplicaciones. Universidad Politécnica de Valencia. Servicio de Publicaciones, 1999.
[8] M. Garcia-Sanz and C. H. Houpis, Wind Energy Systems: Control Engineering Design. CRC Press, 2012.
[9] A. H. Ahmadi and S. K. Y. Nikravesh, “Robust Smith Predictor (RSP),” in 2016 24th Iranian Conference on Electrical Engineering (ICEE), 2016, pp. 1510–1515.
[10] V. M. Alfaro and R. Vilanova, Model-Reference Robust Tuning of PID Controllers. Cham: Springer International Publishing, 2016.
[11] F. N. Deniz and N. Tan, “A Model Identification Method for Tuning of PID Controller in a Smith Predictor Structure,” IFAC-Pap., vol. 49, no. 10, pp. 13–18, Jan. 2016.
[12] F. S. S. de Oliveira, F. O. Souza, and R. M. Palhares, “PID Tuning for Time-Varying Delay Systems Based on Modified Smith Predictor 11This work has been supported by the Brazilian agencies CAPES, CNPq, and FAPEMIG.,” IFAC-Pap., vol. 50, no. 1, pp. 1269–1274, Jul. 2017.
[13] M. Garcia-Sanz and J. G. Guillen, “Smith predictor for uncertain systems in the QFT framework,” in Progress in system and robot analysis and control design, Springer, London, 1999, pp. 239–250.
[14] M. Garcia-Sanz, Robust Control Engineering: Practical QFT Solutions. CRC Press, 2017.
[15] M. G. Martínez, “Síntesis de controladores robustos mediante el análisis de la compatibilidad de especificaciones e incertidumbre,” http://purl.org/dc/dcmitype/Text, Universidad Pública de Navarra, 2001.
[16] C. H. Houpis, S. N. Sheldon, and J. J. D’Azzo, Linear Control System Analysis and Design: Fifth Edition, Revised and Expanded. CRC Press, 2003.
[17] N. Cohen, Y. Chait, O. Yaniv, and C. Borghesani, “Stability analysis using Nichols charts,” Int. J. Robust Nonlinear Control, vol. 4, no. 1, pp. 3–20, 1994.
[18] J. Elso, M. Gil-Martinez, and M. Garcia-Sanz, “Quantitative feedback control for multivariable model matching and disturbance rejection,” Int. J. Robust Nonlinear Control, vol. 27, no. 1, pp. 121–134, Jan. 2017.
[19] M. Gil-Martínez and M. García-Sanz, “Simultaneous meeting of robust control specifications in QFT,” Int. J. Robust Nonlinear Control, vol. 13, no. 7, pp. 643–656, 2003.
[20] Y. Chait and O. Yaniv, “Multi-input/single-output computer-aided control design using the quantitative feedback theory,” Int. J. Robust Nonlinear Control, vol. 3, no. 1, pp. 47–54, Jan. 1993.
[21] A. Visioli, Practical PID Control. London: Springer-Verlag, 2006.